Slopes on the Earth can be natural or man-made. In the construction of highways, railways, earth dams, and river-training works, these are always required. The Geotechnical Engineer is always concerned about the slope stability because the failure would result in loss of life and property.
Slope Stability : Stability of Slope is a critical factor to consider while designing and building earth dams. A natural slope’s stability is also important. The consequences of a slope failure are frequently catastrophic, resulting in the loss of significant property and many lives.
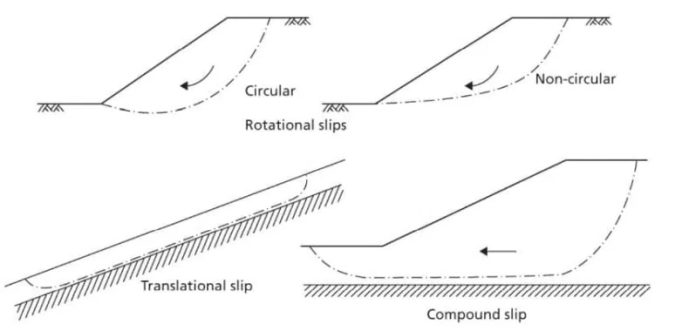
A landslide occurs when an earth slope fails. Gravitational forces and forces caused by water seepage in the soil mass, progressive disintegration of the soil mass structure, and excavation near the base are all major causes of earth slope failure. Earth slope failure can occur slowly or suddenly as a result of slides.
Classification of Slopes
Slope classification can be broadly classified as follows :
Man-made Slopes :
Man-made slopes are the slopes of earth structures that result from human construction activity. Man-made slopes include the sides of cuttings, the slopes of embankments built for roads, railway lines, canals, and the slopes of earth dams built to store water.
Natural Slopes :
Natural slopes are formed by the continuous process of erosion and deposition by natural agencies. Natural slopes include river banks and hill sides, to name a few.
Get more explanation on Natural Slopes at GATE Online Coaching
Whether the slope may be natural or man-made, it has to be among the following:
- Finite Slope
- Infinite Slope
- Homogeneous Slope
- Non-homogeneous Slope
Finite Slope :

The extent of finite slopes is limited. If the slope’s transverse extent is less than the depth of the failure zone, it is called finite. Finite slopes include earthen dams and embankment slopes, to name a few.
Infinite Slope :

The term “infinite slope” refers to a constant slope with an infinite length. This is demonstrated by the long slope of a mountain’s face. If the transverse extent of a slope is greater than the depth of the failure zone, it is called an infinite slope.
Homogeneous Slope :
Within the zone of failure, a slope is called homogeneous if it is made of more or less the same material.
Non-homogeneous Slope :
A slope is called non-homogeneous if it is made up of more than one earth material or if the failure surface passes through two or more zones of different
Causes of Slope Failure
The following are the major factors that contribute to slope instability and failure :
- Gravitational force.
- The force of seepage water.
- Erosion of the slopes surface caused by flowing water.
- A sudden drop in the level of water adjacent to the slope.
- Earthquake-related forces.
All of the forces listed above have the effect of causing soil to move from high to low points. The component of gravity that acts in the direction of probable motion is the most important of these forces. Although the various effects of flowing or seeping water are widely recognized as critical in stability issues, these effects are frequently overlooked. It is a fact that seepage within a soil mass causes seepage forces that have a much greater impact than most people realize.
Slope Stability Analysis & Assumptions
The soil mass must be resistant to any conceivable surface failure on the slope. Although methods based on the theory of elasticity or plasticity are becoming more popular, limiting equilibrium is still the most common method.
Limiting equilibrium methods are statically indeterminate. Because the stress-strain relationships along the assumed surface are unknown, assumptions must be made to make the system statistically determinate and easy to analyse using the equation of equilibrium.
Get more explanation on causes of Slope Failure at Online Courses for PSU Exams
Assumptions of Stability of Slope
- It is assumed that the stress system is two-dimensional. Stresses in the third direction (perpendicular to the soil mass section) are assumed to be zero.
- The Coulomb equation for shear strength is assumed to be valid, and the strength parameters C and Φ are known.
- It’s also assumed that seepage conditions and water levels are known, and that the pore water pressure can be calculated.
- Plastic failure conditions are assumed to be met along the critical surface. In other words, shearing strains are large enough at all points of the critical surface to mobilize all available shear strength.
- Additional assumptions about the magnitude and distribution of forces along various planes are made depending on the method of analysis.
The resultant of all the actuating forces attempting to cause the failure is determined in the slope stability analysis. A determination of the available shear strength is also made. The available resisting forces and actuating forces are used to calculate the slope’s factor of safety.
Factor of Safety in Stability Analysis
In stability analysis two types of factor of safety are most common used :
- Factor of safety with respect to shearing strength
- Factor of safety with respect to cohesion. This is termed the factor of safety with respect to height.
Let,
Fs = factor of safety with respect to strength
Fᴄ = factor of safety with respect to cohesion
Fʜ = factor of safety with respect to height
FΦ = factor of safety with respect to friction
C’m = mobilized cohesion m
Φ’m = mobilized angle of friction
τ = average value of mobilized shearing strength
s = maximum shearing strength
In terms of shearing strength, the factor of safety, Fs, can be written as
The mobilized shear strength at each point on a failure surface can be written as :
or,
where,
&
In reality, shearing resistance (mobilized value of shearing strength) does not develop in the same way at all points on a failing surface.
The shearing strains vary a lot, and the shearing stress isn’t always consistent. However, in average conditions, the above expression is correct.
If the safety factors for cohesion and friction are different, we can write the mobilised shearing resistance equation as follows :
It will be shown later that Fᴄ is dependent on the slope’s height. As a result, the factor of safety with respect to cohesion can be renamed the factor of safety with respect to height.
The ratio between the critical height and the actual height is denoted by the Fʜ. The critical height is the highest point at which a slope can be considered stable. From the above equation we can write,
where FΦ is arbitrarily taken equal to unity.